Answer:
The excluded values of x for
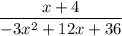 are:
are:
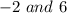
Explanation:
We are given a rational expression as follows:
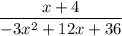
We know that the excluded value of a rational expression are the possible values of x which makes the denominator of the rational expression equal to zero i.e. these are the zeros of the denominator.
The denominator could also be factorized as follows:
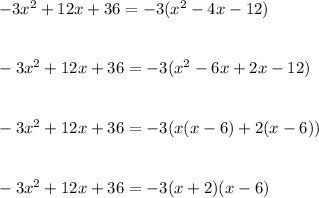
i.e. the zeros of the expression are:
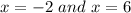
Hence, the excluded values are:
-2 and 6