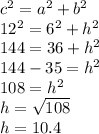Question

What is the height of an equilateral triangle with sides that are 12 cm long? Round to the nearest tenth. (1 point)

Answer 1

The height of an equilateral triangle can be found using the formula h = √3 / 2 × a. Therefore, when we plug in 12 for a, we get that the height of the equilateral triangle is 10.4 centimeters.

Answer 2

10.4 cm

Explanation:

Looking at the triangle in the attached pic, we can see that the right, smaller triangle is a right triangle on which we can apply the pythagorean theorem to solve for the height, h.

Pythagorean theorem tells us
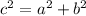`c^2=a^2+b^2` where c is the hypotenuse (12 in our case) and a & b are the two legs (6 and h are the respective lengths).

So let's solve for h:

`c^2=a^2+b^2\\12^2=6^2+h^2\\144=36+h^2\\144-35=h^2\\108=h^2\\h=√(108) \\h=10.4`