Question

At an interest rate of 8% compounded annually, how long will it take to double the following investments?

$50
$500
$1700

Answer 1

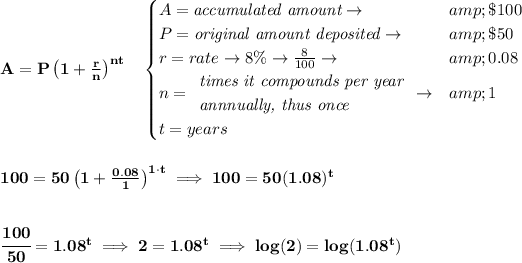
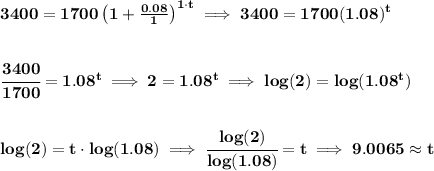let's see, if the first one has a Principal of $50, when it doubles the accumulated amount will then be $100,

recall your logarithm rules for an exponential,


`\bf \textit{Logarithm of exponentials}\\\\ log_{{ a}}\left( x^{{ b}} \right)\implies {{ b}}\cdot log_{{ a}}(x)\\\\ -------------------------------\\\\ \qquad \textit{Compound Interest Earned Amount} \\\\`


`\bf A=P\left(1+(r)/(n)\right)^(nt) \quad \begin{cases} A=\textit{accumulated amount}\to &\$100\\ P=\textit{original amount deposited}\to &\$50\\ r=rate\to 8\%\to (8)/(100)\to &0.08\\ n= \begin{array}{llll} \textit{times it compounds per year}\\ \textit{annnually, thus once} \end{array}\to &1\\ t=years \end{cases} \\\\\\ 100=50\left(1+(0.08)/(1)\right)^(1\cdot t)\implies 100=50(1.08)^t \\\\\\ \cfrac{100}{50}=1.08^t\implies 2=1.08^t\implies log(2)=log(1.08^t) \\\\\\`


`\bf log(2)=t\cdot log(1.08)\implies \cfrac{log(2)}{log(1.08)}=t\implies 9.0065\approx t\\\\ -------------------------------\\\\`

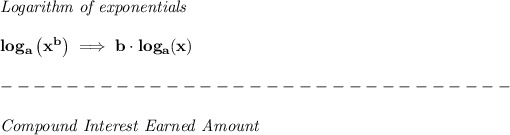
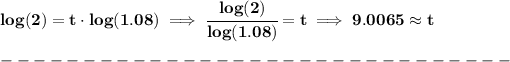
now, for the second amount, if the Principal is 500, the accumulated amount is 1000 when doubled,


`\bf \qquad \textit{Compound Interest Earned Amount} \\\\ A=P\left(1+(r)/(n)\right)^(nt) \quad \begin{cases} A=\textit{accumulated amount}\to &\$1000\\ P=\textit{original amount deposited}\to &\$500\\ r=rate\to 8\%\to (8)/(100)\to &0.08\\ n= \begin{array}{llll} \textit{times it compounds per year}\\ \textit{annnually, thus once} \end{array}\to &1\\ t=years \end{cases} \\\\\\ 1000=500\left(1+(0.08)/(1)\right)^(1\cdot t)\implies 1000=500(1.08)^t \\\\\\`


`\bf \cfrac{1000}{500}=1.08^t\implies 2=1.08^t\implies log(2)=log(1.08^t) \\\\\\ log(2)=t\cdot log(1.08)\implies \cfrac{log(2)}{log(1.08)}=t\implies 9.0065\approx t\\\\ -------------------------------`

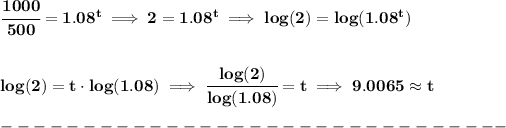
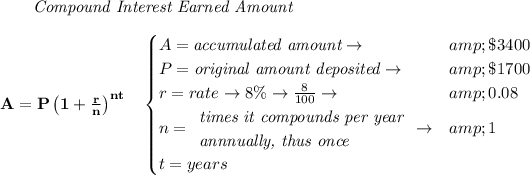
now, for the last, Principal is 1700, amount is then 3400,


`\bf \qquad \textit{Compound Interest Earned Amount} \\\\ A=P\left(1+(r)/(n)\right)^(nt) \quad \begin{cases} A=\textit{accumulated amount}\to &\$3400\\ P=\textit{original amount deposited}\to &\$1700\\ r=rate\to 8\%\to (8)/(100)\to &0.08\\ n= \begin{array}{llll} \textit{times it compounds per year}\\ \textit{annnually, thus once} \end{array}\to &1\\ t=years \end{cases}`


`\bf 3400=1700\left(1+(0.08)/(1)\right)^(1\cdot t)\implies 3400=1700(1.08)^t \\\\\\ \cfrac{3400}{1700}=1.08^t\implies 2=1.08^t\implies log(2)=log(1.08^t) \\\\\\ log(2)=t\cdot log(1.08)\implies \cfrac{log(2)}{log(1.08)}=t\implies 9.0065\approx t`