Question

Which function in vertex form is equivalent to f(x) = x2 + 8 – 16x?

A. f(x) = (x – 8)2 – 56
B. f(x) = (x – 4)2 + 0
C. f(x) = (x + 8)2 – 72
D.f(x) = (x + 4)2 – 32

Answer 1

The answer would be (f(x) = (x – 8)2 – 56)

Answer 2

Answer: The correct option is A.

Step-by-step explanation:

The given function is,

`f(x)=x^2+8-16x`

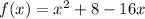
Rewrite the above function.

`f(x)=(x^2-16x)+8`

To make the perfect square we add and subtract the square of
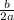`(b)/(2a)`, where b is coefficient of x and a is the coefficient of
`x^2`.

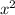Since a=1 and b = -16, So we will add and subtract he square of -8.

`f(x)=(x^2-16x+(-8)^2)+8-(-8)^2`

`f(x)=(x^2-16x+(8)^2)+8-64`

Using
`(a-b)^2=a^2-2ab+b^2`

`f(x)=(x-8)^2)-56`

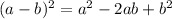
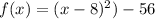
Therefore, the correct option is A.