
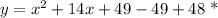


*
Completing the square:
The whole premise behind 'completing the square' was to isolate the x variable by itself in the form of a quadratic function. To understand this concept, we need to look back at the whole idea that when expanding a binomial with degree 2, we will get a predictable pattern specifically:
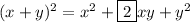
This allowed us to then create a new concept (completing the square), where we attempt to revert back to our simplest form. To understand more about the history and proof, you can search up: "Completing the square".