Question

Find the radius of a circle in which an inscribed square has a side of 8 inches. x =

Answer 1

I know this answer because I am like that 4 sqrt 2

Answer 2

The radius is 5.65 inches.

Explanation:

A diagonal of an inscribed square, is also the hypotenuse of an isosceles right triangle with two sides of the square as legs and it is also the diameter of the circle.

Here, the side of the square is 8 inches.

We have to find the diagonal with both legs 8 inches.

According to Pythagoras theorem, let the diagonal or hypotenuse be x

`8^(2) +8^(2) =x^(2)`

=>
`64+64=x^(2)`

=>
`x^(2) =128`

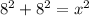
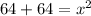
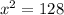
x = √128

x = 11.31

So, this is the diameter of the circle.

The radius will be
`11.31/2=5.65` inches

Hence, the radius is 5.65 inches.