Question

The graph shows the number of albums sold as a function of the number of albums produced.

Which statement is true?



A. As the number of albums produced increases without bound, the number of albums sold increases without bound.

B. As the number of albums sold increases without bound, the number of albums produced decreases without bound.

C. As the number of albums produced decreases, the number of albums sold increases without bound.

D. There is no limit to the number of albums produced, but there is a limit to the number of albums sold.

Answer 1

A. As the number of albums produced increases without bound, the number of albums sold increases without bound.

Explanation:

Answer 2

Answer with explanation:

→→→If you will look at the graph of the function ,which is number of albums produced which is on x axis, and number of album sold which is on y axis,as value of x increases , the value of y increases.

→→To prove this , we will use the concept of increasing and decreasing function.

At,
`x_(1)=3,y_(1)=1000`

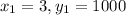
And,
`x_(2)=5, y_(2)=2750`

By the definition of Strictly increasing function if ,

`x_(2)>x_(1)\\\\{\text{then}},y_(2)>y_(1)`

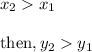
Here, 5>3→→2,750>1,000

So,⇒⇒ the given function which is the relationship between, number of albums produced and number of albums sold , is an increasing function.

Also, if you will look at the graph, there is no upper bound on x (number of albums produced) as well as no upper bound on y ( number of albums sold.)

Option A: As the number of albums produced increases without bound, the number of albums sold increases without bound.