Given
Ladder height = 3 foot
Initisl Velocity = 45 ft/sec
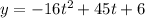
Find
(a) How high it go before returning to the ground.
(b) How long does it take the grapefruit to hit the ground.
Step-by-step explanation
We maximize y , to find critical point
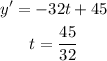
It is a maxima as the given curve is a downward facing parabola.
Maximum Value is
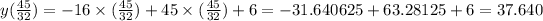
Final Answer
37.640