Question

A girl throws a ball vertically downward at 10m/s from the roof of a building 20m high. Howlong will it take the ball to reach the ground?

Answer 1

1.24 seconds

Step-by-step explanation:

To answer the question we will use the following equation:

`y_f=y_i+v_it+(1)/(2)at^2`

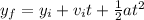
Where:

yf is the final height of the ball, so it is 0m

yi is the initial height of the ball, yi = 20 m

vi is the initial velocity which is vi = -10 m/s because it is downward

a is the acceleration due to gravity a = 9.8 m/s²

t is the time.

So, replacing the values, we get:

`\begin{gathered} 0=20-10t+(1)/(2)(-9.8)t^2 \\ 0=20-10t-4.9t^2 \\ -4.9t^2-10t+20=0 \\ \text{Then} \\ a=-4.9 \\ b=-10 \\ c=20 \end{gathered}`

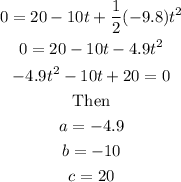
To find the time, we need to solve this equation. So, using the quadratic formula, we get:

`\begin{gathered} t=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a} \\ t=\frac{-(-10)\pm\sqrt[]{(-10)^2_{}-4(-4.9)(20)}}{2(-4.9)} \\ t=\frac{10\pm\sqrt[]{100+392}}{-9.8} \\ t=(10\pm22.18)/(-9.8) \end{gathered}`

Therefore, the values for t are:

`\begin{gathered} t=(10+22.19)/(-9.8)=-3.28 \\ t=(10-22.19)/(-9.8)=1.24 \end{gathered}`

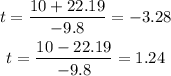
Since t = -3.28 doesn't have sense here, the answer is 1.24 seconds.

The ball reaches the ground after 1.24 seconds.