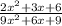Question 1:
Given the three zeros

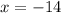
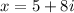
The other zero will be the conjugate pair of
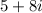
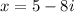
Writing these zeros in factorized form of f(x) gives:
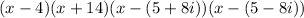
→ Multipy out the first two brackets to get
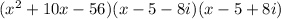
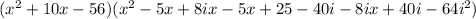
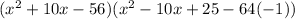
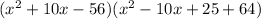
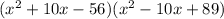
→ Multiply out further
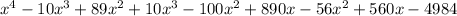

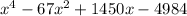
Answer: C
-------------------------------------------------------------------------------------------------------------
Question 2
Given
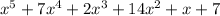
Collecting two terms together to get
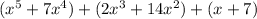
![[x^4(x+7)]+[2x^2(x+7)]+[x+7]](https://img.qammunity.org/2018/formulas/mathematics/high-school/4gzp4gb8r03ia006ildvpl7hy35tua9kgo.png)
Notice that each group has common factor
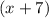
Factorise the common factor gives
![(x+7)[x^4+2x^2+1]](https://img.qammunity.org/2018/formulas/mathematics/high-school/2vmep8bebbsfj6lwfvfmtsd3pzftn4nb3x.png)
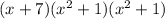
The zeros are
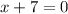
and four of
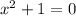
The zero
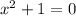
will give an imaginary results
So, f(x) has one real root and four imaginary root
Answer: B
---------------------------------------------------------------------------------------------------------------
Question 3
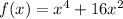
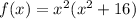
The zeros are
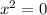
and
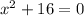
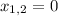
and
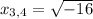
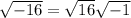
which gives two answers:
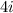
and
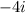
So the factorized form is
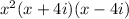
Answer: C
----------------------------------------------------------------------------------------------------------------
Question 4
If

is one of the zeros of

then the other zero would be to conjugate pair of

which is
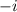
Answer: B
-----------------------------------------------------------------------------------------------------------
Question 5
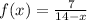
The denominator must not equal zero because we can't divide a quantity by zero (undefined result)
So, we need to find the value of

that would make the denominator equal to zero
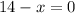
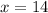
Answer: A
-------------------------------------------------------------------------------------------------------------
Question 6
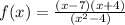
To find vertical asymptote, set the denominator to zero then find 'x'
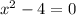

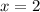
and

Answer: A
----------------------------------------------------------------------------------------------------------
Question 7
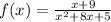
The degree of the denominator is higher than the numerator's, hence the horizontal asymptote is y = 0
------------------------------------------------------------------------------------------------------------
Question 8
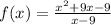
The degree of numerator is higher than the degree of the denominator, hence no horizontal asymptote
------------------------------------------------------------------------------------------------------------
Question 9
To have a horizontal asymptote,
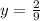
, both numerator and denominator must be on the same polynomial degree, and the leading degree have coefficient of 2 and 9
Example: