Solution
ou have a revenue function and you have a cost function and you want a profit function.
profit = revenue minus cost, so if you subtract the cost function from the revenue function, you will get the profit function.
your revenue function is y = -23 * x^2 + 149000 * x.
your cost function is y = -19 * x^2 + 61000 * x + 18324.
your profit function will be y = -23 * x^2 + 149000 * x - (-19 * x^2 + 61000 * x + 18324.).
simplify to get y = -23 * x^2 + 149000 * x - 19 * x^2 - 61000 * x - 18324.
combine like terms to get y = -4 * x^2 + 88000 * x - 18324.
that's your profit function.
you want to know when your profit will be maximum.
since this is a quadratic function that's in standard form, you get:
a = -4
b = 88000
c = -18324.
the maximum profit will be when x = -b / 2a.
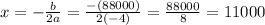
when x = 11000, y =
![undefined]()