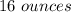Given that:
- Marie is making a soup that contains 80 total ounces of vegetables.
- There are 4 times as many ounces of celery as there are carrots.
Let be "x" the number of ounces of celery in the soup and "y" the number of ounces of carrots in the soup.
You can set up the following System of Equations using the data given in the exercise:

Notice that the first equation represents the total ounces of vegetables in the soup, and the second equation represents the number of ounces of celery in the soup.
You can find the value of "y" using the Substitution Method:
1. Substitute the second equation into the first equation:
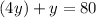
2. Solve for "y":
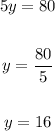
Hence, the answer is:
Option D: