Question

What are the focus and the directrix of the graph of x = 1/24y^2?

focus (0,-6), directrix x= 6
focus (6,0) directrix x= -6
focus (0,6) directrix y= -6
focus (-6,0) directrix y- 6

Answer 1

The answer is:

B. focus (6, 0), directrix x=-6

Answer 2

`\bf \textit{parabola vertex form with focus point distance}\\\\ \begin{array}{llll} \boxed{(y-{{ k}})^2=4{{ p}}(x-{{ h}})} \\\\ (x-{{ h}})^2=4{{ p}}(y-{{ k}}) \end{array} \qquad \begin{array}{llll} vertex\ ({{ h}},{{ k}})\\\\ {{ p}}=\textit{distance from vertex to }\\ \qquad \textit{ focus or directrix} \end{array}\\\\ -------------------------------\\\\ x=\cfrac{1}{24}y^2\implies 24x=y^2\implies 24(x-0)=(y-0)^2 \\\\\\ 4(\stackrel{p}{6})(x-\stackrel{h}{0})=(y-\stackrel{k}{0})^2`

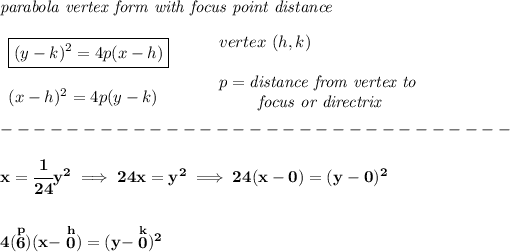
now, notice, the "y" is the squared variable, meaning is a parabola opening sideways.

the leading term's coefficient, or even "p" sign is positive, meaning the parabola is opening to the right-hand-side.

now, notice the vertex h,k, is at the origin.

to get the focus, we then move "p" units to the right, where it opens, and we will end up at (6,0).

to get the directrix, we move in opposite direction, to the left, "p" units as well, and we will end up at x = -6.