Given the triangle shown in the exercise, you know that the entrance to the path is halfway between your house and your friend's house.
a. You need to remember that, by definition, an Angle Bisector is a line that divides an angle into two equal parts.
In this case, if the path bisects the angle formed by Oak and Maple Streets, you know that the two angles obtained are two congruent angles. According to the Angle Bisector Theorem, that line also divides the opposite side into two segments that are proportional to the other sides.
Look at the picture shown below:
You can set up that:
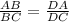
However, this does not specify that the distances AD and DC are equal. Therefore, you cannot determine if you and your friend live the same distance from the school by knowing that DB (the path) bisects the indicated angle.
b. If the path is perpendicular to Birch Street, that means that the lines DB and AC are perpendicular.
By definition, a Perpendicular Bisector is a line that passes through the midpoint of a segment and formed an angle that measures 90 degrees.
Then, if DB is a Perpendicular Bisector, according to the theorem:

Therefore, you can conclude that you and your friend live the same distance from the school.
c. Hence, you can conclude that the triangle is isosceles if:

The triangle is isosceles if the legs of the triangle are equal in length.
Therefore, the answers are:
a. No, because you know that it divides the angle into two equal angles, but there is not enough information to determine that the distance between the school and your house, and the distance between the school and your friend's house are equal.
b. Yes, because a Perpendicular Bisector is a line that passes through the midpoint and indicates that the legs of the triangle are equal in length.
c. The triangle is isosceles if the legs of the triangle are equal in length.