Question

In the kite, find the value of w x y and z

Answer 1

• w = 4cm

,

• x = 90 degrees

,

• y = 39 degrees

,

• z = 18 degrees

Explanation:

The given figure is a kite.

A kite is made up of two isosceles triangles joined base to base. Its diagonals are not equal but the longer one cuts the shorter in half at 90 degrees.

Some of the properties of a kite that will be useful in solving these problems are listed below.

• I. Kite has 2 diagonals that intersect each other at right angles.

,

• II. The longer diagonal divides the shorter diagonal into two equal parts.

,

• III. A kite is symmetrical. So it has two opposite and equal angles.

By property I above, the value of x is 90 degrees.

By property II above, the length of w = 4cm.

In the smaller triangle:

`\begin{gathered} 51\degree+51\degree+2y=180 \\ y=180-102 \\ y=(78)/(2) \\ y=39\degree \end{gathered}`

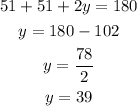
The value of y is 39 degrees.

Similarly, in the larger Isosceles triangle:

`\begin{gathered} 72\degree+72\degree+2z=180\degree \\ 2z=180\degree-(72+72) \\ 2z=180-144 \\ 2z=36\degree \\ z=(36\degree)/(2) \\ z=18\degree \end{gathered}`

The value of z is 18.