Question

F(x)=-2(0.5)x domain:(0,1,2)

Answer 1

Given the below function

f(x) = -2(0.5)^x

Domain: 0, 1 ,2

Let the value of the domain = x

To find the function, substitute the value of into the above function

`\begin{gathered} f(x)=-2(0.5)^x \\ \text{Let x = }0 \\ f(0)\text{ = -2(0}.5)^0 \\ \text{ According to the law of indicies, anything raised to the power of 0 = 1} \\ f(0)\text{ = -2 x 1} \\ f(0)\text{ = -2} \\ \text{When x = 1} \\ f(1)=-2(0.5)^1 \\ f(1)\text{ = -2 x 0.5} \\ f(1)\text{ = -1} \\ \text{When x = 2} \\ f(2)=-2(0.5)^2 \\ f(2)\text{ = }-2\text{ x 0.25} \\ f(2)\text{ = - 0.5} \end{gathered}`

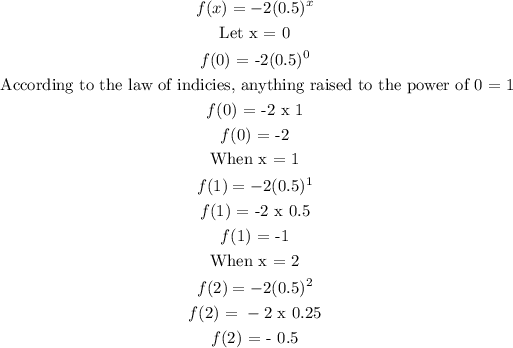
From the above solution, we can now draw out our table

x 0 1 2

f(x) -2 -1 -0.5

Let us graph the above pairs