By Stokes' theorem,

where
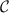
is the circular boundary of the hemisphere
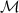
in the

-

plane. We can parameterize the boundary via the "standard" choice of polar coordinates, setting
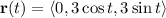
where
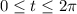
. Then the line integral is

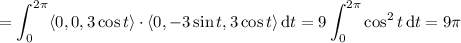
We can check this result by evaluating the equivalent surface integral. We have
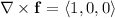
and we can parameterize
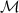
by
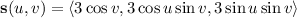
so that
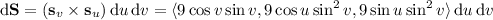
where

and
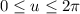
. Then,
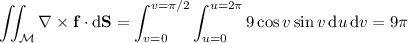
as expected.