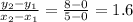Answer:
The answer is below
Explanation:
The equation of a straight line is given by:
y = mx + b; where y and x are variables, b is the y intercept (i.e. value of y when x is zero), m is the slope of the line.
The slope of a line passing through the points
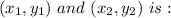
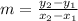
Let the point where the two lines intersect be the origin (0, 0).
Line 1 passes through (0, 0) and (8, 5). Hence:
slope of line 1 =
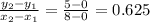
Line 2 passes through (0, 0) and (5, 8). Hence:
slope of line 2 =