Answer:
x = 437.3 ft
Explanation:
Right Triangles
In right triangles, where one of its internal angles measures 90°, the trigonometric ratios are satisfied.
We have completed the figure below with the missing internal angle A that measures A = 90° - 29° = 61° because the lines marked with an arrow are parallel.
Given the internal angle A, we can relate the unknown side of length x with the known side length of 500 ft, the hypotenuse of the triangle. We use the sine ratio:
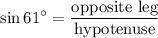
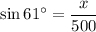
Solving for x:
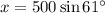
Calculating:
x = 437.3 ft