To see what function corresponds to the table we can start by trial and error.
But we can have a hint to make it easier. We know that a number to the power of 0 will be 1. The table shows that, when x (the expoonent) is 0, we obtain a value of y = 6. This means that in the function, the number that has the x exponent should be multiplied by 6, so when it is to the power of 0 we obtain:
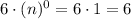
With n being any number.
With this we can say that the correct answer is the third option. Let's prove it:
When x = -1, y = 12:

When x = 0, y = 6:
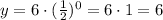
When x = 1, y = 3:
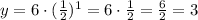
When x = 2, y = 3/2:
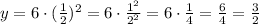
This proves the correct answer is the third option. It satisfied each point of the table.