Question

Ben and Joel are raising money for their class trip by selling 12 rolls of solid paper and 9 rolls of printed paper. Find the cost of each type of wrapping paper.

Answer 1

Explanation:

Step 1. To solve this problem we need to set up a system of equations.

Our variables will be:

`\begin{gathered} x\rightarrow cost\text{ of a roll of solid paper} \\ y\rightarrow cost\text{ of a roll of printed paper} \end{gathered}`

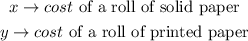
Step 2. Our first equation is given by the money that Ben raised. Since sold 12 rolls of solid paper, and 9 rolls of printed paper and the amount raised from that is 43.50, the equation that describes this is:

`12x+9y=43.50`

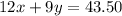
Step 3. Similarly, since Joel sold 8 rolls of solid paper and 15 rolls of printed paper and the amount that he raised was 51.50, the equations that describe this is:

`8x+15y=51.50`

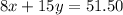
Step 4. To find the value of x and y, we need to solve the system of equations. We will use the elimination method, which consists of modifying the equations so that when we add or subtract them one variable is eliminated.

We are going to eliminate variable y, so we multiply the first equation by 5/3:

`\begin{gathered} (5)/(3)(12x+9y=43.50) \\ \downarrow \\ 20x+15y=72.5 \end{gathered}`

Step 5. Our system of equations now is:

`\begin{gathered} 1st\text{ equation: } \\ 20x+15y=72.5 \\ 2nd\text{ equation:} \\ 8x+15y=51.50 \end{gathered}`

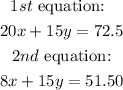
Step 6. Subtracting the second equation from the first equation:

The resulting equation is:

`\begin{gathered} 12x=21 \\ \downarrow \\ x=21/12 \\ \downarrow \\ \boxed{x=1.75}cost\text{ of a roll of solid paper} \end{gathered}`

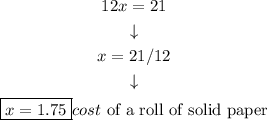
Step 7. Using the x value to find the value of y:

`\begin{gathered} 12x+9y=43.50 \\ \downarrow \\ 12(1.75)+9y=43.50 \\ \downarrow \\ 21+9y=43.50 \\ \downarrow \\ 9y=43.5-21 \\ \downarrow \\ y=22.5/9 \\ \downarrow \\ \boxed{y=2.5}cost\text{ of a roll of printed paper} \end{gathered}`

Answer:

$2.5 is the cost of a roll of printed paper

$1.75 is the cost of a roll of solid paper