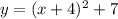Answer:
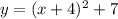
Explanation:
We are given that vertex of parabola is (-4,7).
We have to find the equation of parabola in vertex form which passing through the point (-3,8).
We know that general equation of parabola in vertex form
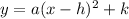
Where vertex is (h,k).
Substitute the values
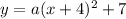
Parabola passing through the point (-3,8) then we get
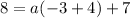
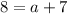
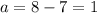
Substitute the value then we get
Equation of parabola in vertex form