Solution: A quadrilateral RSTU whose vertices are R(−4, 1) , S(4, −1) , T(3, −6) , and U(−5, −4).
RS =

ST=
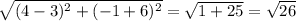
TU=
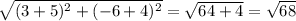
UR =

→RS=TU, and ST=UR⇒ Opposite sides are equal.
Slope of RS =
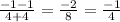
Slope of TS=
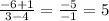
Slope of RS × Slope of TS = -1/4 × 5 = -5/4 ≠ -1, So lines are not perpendicular.
∴ quadrilateral RSTU is not a rectangle.