The relationship between x and y is represent as:
Since, the relationship is linear.
The standard form of equation of line is:
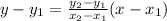
Consider any two set x and y values from the given relationship.
Let (-2, 10) and (-1,7.5)
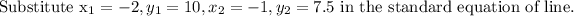
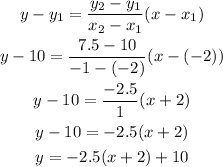
The equation of the linear relationship between x and y is:
y = -2.5(x + 2) + 10
Now, to check that the point (9, -17.5) lies on the represented relationship between x and y
Substitute x = 9 and y = -17.5 in the equation y = -2.5(x + 2) + 10
y = -2.5(x + 2) + 10
-17.5 = -2.5(9 + 2) + 10
-17.5 = -2.5(11) + 10
-17.5 = -27.5 + 10
-17.5 = -17.5
Thus, LHS = RHS
Hence the point (9, -17.5) lie on the given linear relationship between x and y.
Answer: The point (9, -17.5) lie on the given linear relationship between x and y.