Question

The area of a sector of a circle is 23.2 in2. If the diameter ofthe circle is 24 in., what is the value of the missing degree of thesector? (0)

Answer 1

The area of the sector of a circle is expressed as

`\begin{gathered} Area_(sector)=(\theta)/(360)*\pi r^2\text{ ---- equation 1} \\ where \\ \theta\Rightarrow angle\text{ subtended by the sector} \\ r\Rightarrow radius\text{ of the circle} \end{gathered}`

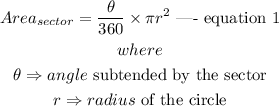
Given that the area of the sector of the circle is 23.2 square inches, and the diameter of the circle is 24 inches, to calculate the missing degree of the sector,

step 1: Evaluate the radius r of the circle.

The radius of the circle is expressed as

`radius=(diameter)/(2)`

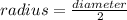
Thus, the radius of the circle is evaluated to be

`\begin{gathered} r=(24)/(2) \\ =12\text{ inches} \end{gathered}`

step 2: Substitute the parameters (area of sector, radius of circle) into equation 1.

Thus, from equation 1

`\begin{gathered} \begin{equation*} Area_(sector)=(\theta)/(360)*\pi r^2 \end{equation*} \\ 23.2=(\theta)/(360)*\pi*12*12 \\ multiply\text{ through by 360} \\ 360(23.2)=360((\theta)/(360)*\pi*12*12) \\ \Rightarrow8352=144\pi*\theta \\ divide\text{ both sides by 144}\pi \\ (8352)/(144\pi)=(144\pi*\theta)/(144\pi) \\ \Rightarrow\theta\text{=18.4619734}\degree \end{gathered}`

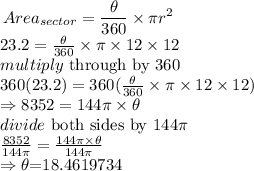
Hence, the value of the missing degree of the sector is 18.4619734°.