Answer:
1,890 is the sum of the first 30 terms of the equation .
Explanation:

if , n = 1

If, n = 2

If, n = 3
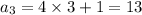
The arithmetic sequence comes out to be:
5, 9, 13........
a = 5
d =
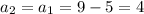
The sum of first 30 terms is given by:
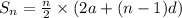
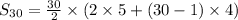

1,890 is the sum of the first 30 terms of the equation .