ANSWER and EXPLANATION
We want to test for symmetry for the given function:
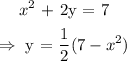
The function can be symmetric:
=> about the y axis
=> about the x axis
=> about the origin
ABOUT Y AXIS
To test for this, we replace x with -x and see if the function is the same as the original:
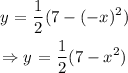
Since the equation is the same as the original, then it is symmetrical about the y axis.
ABOUT X AXIS
To test for this, we replace y with -y and see if the function is the same as the original:

Since this is not the same as the original, it is not symmetrical about the x axis.
ABOUT THE ORIGIN
For this test, we have to replace y with -y and x with -x and then check if the function is the same as the original. It is a combination of the two above.
Since we already saw above that replacing y with -y shows that it is not symmetric about the y axis, we can conclude that it is also not symmetric about the origin.
Therefore, the graph of the equation possesses a symmetry about the y axis.