Step-by-step explanation:
The equation for the x-value of the vertex of a quadratic equation:
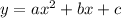
is:
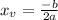
And to find the y-value of the vertex we have to replace its x-value into the quadratic equation.
In this problem we have b = -12 and a = 4
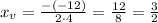
And the y-value:

Answer:
The vertex of this parabola is located at point (3/2, -4)