Question

Find probabilities using combinations and permutations. Emir is handing out 10 invitations to his New Year’s Eve party, 4 of which are printed on blue paper. If Emir randomly hands out 5 invitations before lunch, what is the probability that exactly 2 of the chosen invitations to are printed on blue paper? Write your answer as a decimal rounded to four decimal places.

Answer 1

We have got 10 invitations, 4 are i blue paper, so 6 are not in blue paper.

To find the probability, we will calculate how many combinations are there and how many combination in which exactly 2 out of 5 of the invitations are blue.

The total combinations of the 5 are just 10! over 5!:

`T=(10!)/(5!)=10\cdot9\cdot8\cdot7\cdot6`

The combinations of ecatly 2 blue starts with 4*3/2!, becase initially we have 4 to choose from but the second we have one less. Now we multiply by the same for the non blue, so 6*5*4/3!, and we get:

`B=(4\cdot3)/(2\cdot1)\cdot(6\cdot5\cdot4)/(3\cdot2\cdot1)=2\cdot3\cdot5\cdot4`

The probability is the combinations of the event we want, B, divided by the total combinations, T:

`(B)/(T)=(2\cdot3\cdot5\cdot4)/(10\cdot9\cdot8\cdot7\cdot6)=(5\cdot4)/(10\cdot9\cdot8\cdot7)=(4)/(5\cdot9\cdot8\cdot7)=(1)/(2\cdot9\cdot2\cdot7)=(1)/(252)=0.003968\ldots\approx0.0040`

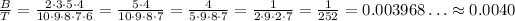
So, the probability that exactly 2 of the choosen invitations are printed in blue is 0.0040.