Question

The stage 2 triangle has side length of one-half.a) Find the height of one of the triangles in stage 2.b) Find the area of one of the triangles in stage 2. Be sure to simplify.

Answer 1

We will have the following:

A) The height of one of the triangles in stage 2 is also one-half.

B) The area would be:

`A=(((1)/(2)b)((1)/(2)h))/(2)\Rightarrow A=(1)/(8)bh`

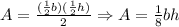
So, the area would be 1/8bh [Since the values of b & h are unknown they remain as variables].

***Explanation***

We can see that at stage 2 each base if each triangle is equivalent to one-half of the triangle at stage 1. From this, we can see then, that "the middle" of the triangle would be given by the interception point of both bases at stage 2, and since the height of the triangle at stage 1 has a measurement of "h" [We name h the height of the triangle] then, the height of each individual triangle at stage 2, will also be one-half of the original.