Question

Rewrite fx) = x + 8x-20 in the form that would most easily help you identifythe zeros of the function.A. f(x) = (x+47 - 20B. f(x) = (x-10)(x+2)C. f(x) = (x+4% -36D. f(x) = (x + 10)(x-2)

Answer 1

The given function is:

`f(x)=x^2+8x-20`

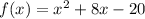
To identify the zeros of the function, substitute x = 0 in each given function if they give the same constant term as in the original function then that function is rewritten of the given function.

First, put x =0 in the original function gives:

`\begin{gathered} f(0)=0^2+8*0-20 \\ f(0)=-20 \end{gathered}`

Now, if the functions given in the option give the same constant -20 after putting x = 0 then that function can be rewritten of the original function.

In option A

Put x = 0, it gives:

`\begin{gathered} f(x)=(x+4)^2-20 \\ f(0)=(0+4)^2-20 \\ f(0)=16-20 \\ f(0)=-4 \end{gathered}`

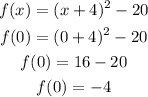
This function gives -4.

Thus, option A is not correct.

In option B,

Put x = 0, it gives:

`\begin{gathered} f(x)=(x-10)(x+2) \\ f(0)=(0-10)(0+2) \\ f(0)=-10*2 \\ f(0)=-20 \end{gathered}`

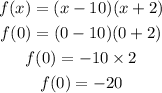
Therefore, option B is correct.

In option C

Put x = 0, it gives:

`\begin{gathered} f(x)=(x+4)^2-36 \\ f(0)=(0+4)^2-36 \\ f(0)=16-36 \\ f(0)=-20 \end{gathered}`

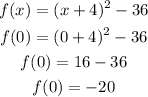
Therefore, option C is also correct.

In option D,

Put x = 0, it gives:

`\begin{gathered} f(x)=(x+10)(x-2) \\ f(0)=(0+10)(0-2)_{} \\ f(0)=10*(-2) \\ f(0)=-20 \end{gathered}`

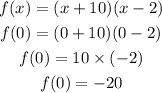
Therefore, option D is also correct.

Hence, the correct options are B, C, D.