Question

20) Why does a vertical line have an undefined slope? What makes this different from a horizontal line with a zero slope?

Answer 1

Let's draw a vertical line on the coordinate plane:

we have that the vertical line passes through the points (x,y_1) and (x,y_2). So if we want to find the slope this would happen:

`m=(y_2-y_1)/(x_2-x_1)=(y_2-y_1)/(x-x)=(y_2-y_1)/(0)_{}`

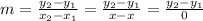
So, we have that a vertical line have undefined slope since the denominator becomes 0 when we calculate the slope.

Now for the horizontal line, we have the following:

If we calculate the slope we get the following:

`m=(y_2-y_1)/(x_2-x_1)=(y-y)/(x_2-x_1)=(0)/(x_2-x_1)=0`

As we can see, the slope of a horizontal line is 0 since the values of the numerator become 0, and that is defined as a real number.