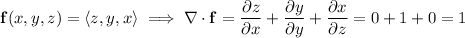
Converting to spherical coordinates, we have
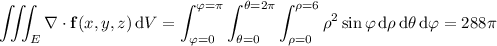
On the other hand, we can parameterize the boundary of
by
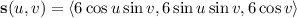
with
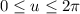
and
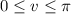
. Now, consider the surface element
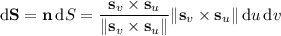
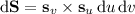
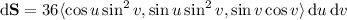
So we have the surface integral - which the divergence theorem says the above triple integral is equal to -

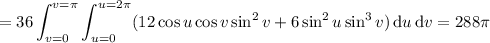
as required.