Question

Jack is selling wristbands and headbands to earn money for camp. He earns $2 for each wristband and $3 for each headband. He wants to earn at least $50. He needs to sell at least 5 wristbands. Which system of inequalities is shown in the graph of the solution below?

• 2x + 3y ≥ 50 x ≥ 5 x ≥ 0 y ≥ 0
•3x + 2y ≥ 50 x ≥ 5 x ≥ 0 y ≥ 0
•2x + 3y ≤ 50 x ≥ 5 x ≥ 0 y ≥ 0
•2x + 3y ≤ 50 x ≤ 5 x ≥ 0 y ≥ 0

Answer 1

Your answer will most likely be a.

Answer 2

The correct option is A.

Explanation:

Let number of sold wristbands and headbands be x and y respectively.

It is given that the jack earns $2 for each wristband and $3 for each headband. So, total revenue is

`T=2x+3y`

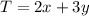
He wants to earn at least $50.

`2x+3y\geq 50`

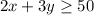
He needs to sell at least 5 wristbands.

`x\geq 5`

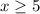
The number of sold wristbands and headbands can not be negative. so,

`x\geq 0,y\geq 0`

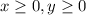
The system of inequalities is

`2x+3y\geq 50`

`x\geq 5`

`x\geq 0,y\geq 0`

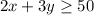
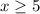
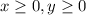
Therefore, correct option is A.