Question

`(1 + 0.12 / 12) { 12 * 1 / 2} - 1 / 0.12 / 12 =`

Answer 1

The answer is:
________________________________________________________
"
`(4829)/(900)` "; or, "5
`(329)/(900)`" ;
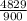
or, write as: "5.366" .
________________________________________________________
Step-by-step explanation:
__________________________________
Given: " (1 + 0.12 ÷ 12)12 * 1 ÷ 2− 1 ÷ 0.12 ÷ 12 = ? " ;
___________________________________________

First, start with the "parentheses" ;
______________________________
"(1 + 0.12 ÷ 12) = ??

Start with the division: " 0.12 ÷ 12 = 0.01 " .

"(1 + 0.01)" = (1.01) .

We have: "1.01 (12)
Given: " (1.01)12 * 1 ÷ 2 − 1 ÷ 0.12 ÷ 12 = ? " ;

So, 1.01 * 12 = 12 .12 .
______________________
" 12.12 * 1 ÷ 2 − 1 ÷ 0.12 ÷ 12 = ? " ;

Note: Using order of operations, treat this problem as:

" [ (12.12 * 1) ÷ 2 ] − [ (1 ÷ 0.12) ÷ 12 ] = ? " ;

→ ( 12.12 ÷ 2 ) − (25/3) ÷ 12 ] = ? " ;

→ ( 6.06) − { (25/3) * 1/12) } = ?

→ ( 6.06) − { ( 25* 1) / (3*12) = ?

→ ( 6.06) − { ( 25* 1) / (3*12) = ?

→ ( 6.06) − (25/36) ;

→ 6
`(6)/(100)` −
`(25)/(36)` ;

→ 6
`(3)/(50)` −
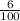
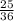
`(25)/(36)` ;

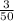
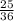
Rewrite " 6
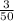
`(3)/(50)` " ; as an improper fraction:

→ " 6
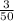
`(3)/(50)` " = "[ (50*6) + 3 ] / 50 =
`(303)/(50)` ;
__________________________________________
→ 6
`(3)/(50)` −
`(25)/(36)` ;

=
`(303)/(50)` −
`(25)/(36)` ;

=
`((303*36)-(25*50))/(50*36) = (10,908-1250)/(1800)` ;

=
`(9658)/(1800)` ;
_________________________________
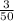
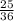
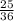
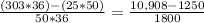
→ Divide EACH SIDE of the "fraction" by "2" ; to simplify:
____________________________________________________
→
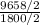
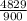
`(9658/2)/(1800/2)` ;
=
`(4829)/(900)` = 5
`(329)/(900)` ; or, write as:

(5 + (329 ÷ 900) = → 5.3655555555555556 .
→ round to: 5.366 .
________________________________________________________