Question

Find the Perimeter of the figure below, composed of a rectangle and a semicircle.Round to the nearest tenths place.108Answer:Submit Answer

Answer 1

The perimeter is the whole sides of the figure. The figure has a rectantangle and an attached semi circle.

The circumfernce of a circle is the perimeter of a circle. The circumference of a circle is

`\text{circumference}=2\pi r`

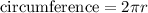
Since the attached part is a semi circle the circumference will be

`\begin{gathered} \text{circumference}=(1)/(2)*2\pi r \\ \text{circumference}=\pi r \\ r=(8)/(2)=4 \\ \text{circumference}=4\pi \end{gathered}`

perimeter of the figure is therefore,

`\begin{gathered} \text{perimeter}=8+10+10+4\pi \\ \text{perimeter}=28+4\pi \\ \text{perimeter}=28+(4*22)/(7) \\ \text{perimeter}=28+(88)/(7) \\ \text{perimeter}=28+12.5714285714 \\ \text{perimeter}=40.5714285714 \\ \text{perimeter}\approx40.6 \end{gathered}`