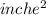Question

A right rectangular prism is 5 inches long, 12 inches wide, and 8 inches tall. The diagonal of the base is 13 inches.

The area of the cross section that is perpendicular to the base and created by a plane that slices the prism through the diagonals of the base and its opposite face is _ ?
square inches.

Answer 1

I think that would be  a rectangle with sides 13 and  8  Which =  104 ins^2

Answer 2

Area of cross section is 104
`\:inche^2`

Explanation:

Given Dimensions of Rectangular prism are Length = 5 inches, Width = 12 inches and Height = 8 inches.

The shape of the cross section that is perpendicular to the base and created by slicing the prism through diagonals of top and bottom is Rectangle.

Length of rectangle = Length of diagonal of base & top = 13 inches

Width of Rectangle = Height of Prism = 8 inches

Area of Cross Sectional Rectangle = Length × Width

= 13 × 8

= 104
`\:inche^2`

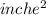
Therefore, Area of cross section is 104
`\:inche^2`