Question

Find the parabola whose minimum is at (−12,−2)(−12,−2) rather than the point given in the book. the parabola's equation is y=x2+ax+by=x2+ax+b, where

Answer 1

The vertex form of the equation of a parabola is given by


`y-k=a(x-h)^2`

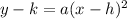
where (h, k) is the vertex of the parabola.

Given that the vertex of the parabola is (-12, -2), the equation of the parabola is given by


`y-(-2)=a(x-(-12))^2 \\ \\ y+2=a(x+12)^2=a(x^2+24x+144)=ax^2+24ax+144a \\ \\ y=ax^2+24ax+114a-2 \\ \\ y=x^2+24x+ (114a-2)/(a)`

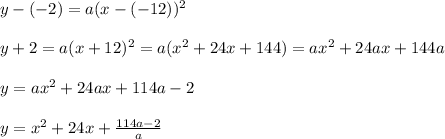
For a = 1,


`y=x^2+24x+112`

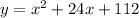The parabola whose minimum is at (−12,−2) is given by the equation
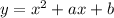`y=x^2+ax+b`, where a = 24 and b = 112.