Question

- Save & Exit CertifyLesson: 13.7 Applications: Quadratic Equat...ISAIAH COVERTQuestion 4 of 17, Step 1 of 14/21CorrectOne integer is 4 more than another. Their product is 165. Find the integers.AnswerHow to enter your answer (opens in new window)KeypadKeyboard Shortcutssmaller integer =larger integer =

Answer 1

Given that,

One integer is 4 more than another.

Their product is 165.

Let the intergers be x and y.

Let x be the larger integer.

we get,

`x=4+y----(1)`
`xy=165----(2)`

Substitute equation (1) in equation (2), we get,

`(4+y)y=165`
`4y+y^2=165`

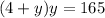
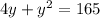
Solving this we get,

`y^2+4y-165=0`

we get,

`y^2+15y-11y-165=0`
`y(y+15)-11(y+15)=0`

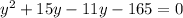
Taking y+15 as common, we get

`(y+15)(y-11)=0`

`y=-15\text{ or }y=11`

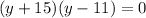
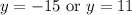
Hence there are two possible solutions

when y=-15, we get x=-11,

And when y=11, we get x=15

Largest integer = -11 or 15

Smallest integer = -15 or 11

Answer is:

Largest integer = -11 or 15

Smallest integer = -15 or 11