Question

What is the difference between the mean and the median of the data set? {22, 8, 10 ,18 ,12, 20} 0 2 4 6

Answer 1

Option A - 0

Explanation:

Given : Data set {22, 8, 10 ,18 ,12, 20}

To find : What is the difference between the mean and the median of the data set?

Solution :

Data set in increasing order {8, 10 ,12,18, 20,22}

Mean is defined as the division of sum of set values by total number of values.

`M=(\sum x)/(n)`

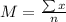
Substitute the values we get,

`M=(8+10+12+18+20+22)/(6)`

`M=(90)/(6)`

`M=15`

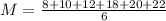
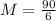
So, The mean of the set is 15.

Median of even numbers is the average of two middle terms.

`m=(3rd+4th)/(2)`

`m=(12+18)/(2)`

`m=(30)/(2)`

`m=15`

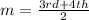
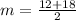
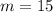
So, The median of the set is 15.

Now, The difference of the mean and the median of the set is

`d=M-m`

`d=15-15`

`d=0`

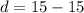
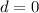
Therefore,Option A is correct.

The difference of the mean and the median of the set is 0.

Answer 2

Answer: The correct option is (A) 0.

Step-by-step explanation: We are given to find the difference between the mean and median of the following data set:

{22, 8, 10 ,18 ,12, 20}.

MEAN: It is the sum of all the values together divided by the number of values in the set.

So, the MEAN of the given data set is

`m=(22+8+10+18+12+20)/(6)\\\\\\\Rightarrow m=(90)/(6)\\\\\Rightarrow m=15.`

MEDIAN: The median of a data set is given by the middle-most value in the data.

If there is an even number of items in the data set, then the median is found by taking the mean of the two middlemost numbers.

Arranging the given data in ascending order, we have

8, 10, 12, 18, 20, 22.

Since there are 6 values, that is even number of values. so the MEDIAN is given by

`M=(12+18)/(2)\\\\\Rightarrow M=(30)/(2)\\\\\Rightarrow M=15.`

Therefore, m = M.

That is, mean and median of the given data are equal.

Hence, the difference is

m - M = 15 - 15 = 0.

Thus, option (A) is CORRECT.