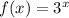Question

Find the exponential function that is the best fit for f(x) defined by the table below.

Answer 1

So we must find an exponential function. The general form of these functions is:

`f(x)=a\cdot b^x`

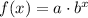
By using the table provided by the question we can find the values of a and b and thus the expression of the function. In this case we can take two pairs of values from the table. On one hand we have that x=1 and f(1)=3, on the other hand we have x=2 and f(2)=9. Using the former formula we can build two equations:

`\begin{gathered} f(1)=3=a\cdot b^1 \\ f(2)=9=a\cdot b^2 \\ \text{Then we have a system of two equations:} \\ 3=a\cdot b \\ 9=a\cdot b^2 \end{gathered}`

Let's use the first one:

`\begin{gathered} 3=a\cdot b \\ b=(3)/(a) \end{gathered}`

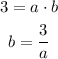
Now we substitute 3/a in place of b in the second equation:

`\begin{gathered} 9=a\cdot b^2 \\ 9=a\cdot((3)/(a))^2 \\ 9=a\cdot(9)/(a^2)=(a\cdot9)/(a\cdot a)=(9)/(a) \\ 9=(9)/(a) \\ a\cdot9=9 \\ a=(9)/(9)=1 \end{gathered}`

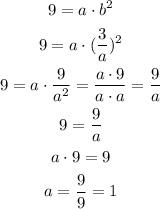
So a=1 and since we found that b=3/a then b=3. Then the exponential function that we are looking for is:

`f(x)=3^x`