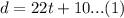Question

John bikes 22km per hour and starts at mile 10. Gwynn bikes 28 km per hour and starts at mile 0. Which system of linear equations represents this situation?

Answer 1

If j is the distance in km John reaches after time t hours, and g is the same for Gwynn, the equations are
j=10+22t and g=28t.
If x is the distance at which they meet the equations form a system from which t, when they meet, can be calculated: 10+22t=28t, 6t=10, t=5/3=1 hour 40 minutes. And they meet at 28×5/3=10+22×5/3=46.67km.

Answer 2

`d=22t+10...(1)`

`d=28t...(2)`

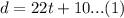
Explanation:

Let t represent the number of hours and d represent the total distance after t hours.

We have been given that John bikes 22 km per hour and starts at mile 10. This means that slope of line will be 22 and y-intercept will be 10. So total distance covered by John in t hours will be:

`d=22t+10...(1)`

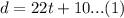
We are also told that Gwynn bikes 28 km per hour and starts at mile 0. This means that slope of line will be 28 and y-intercept will be 0. So total distance covered by Gwynn in t hours will be:

`d=28t+0`

`d=28t...(2)`

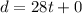
Therefore, our required system of linear equations will be:

`d=22t+10...(1)`

`d=28t...(2)`