Question

The specific heat of silver (ag) is 0.245 j/ºc g. determine the energy required to raise the temperature of 350 g of ag from 293 k to 400 k.

Answer 1

9180 Joules First determine how many degrees the temperature is being changed. 400 K - 293 K = 107 K Since the units K and C have the same magnitude (they just have a different 0 point). We can freely exchange them when merely speaking of differences and not absolute values. So we can express the specific heat of silver as 0.245 J/ÂşC g or 0.245 J/K g. In any case, we now have 3 values with the units J/K g, g, and K. We desire a result with the units J. So it's a simple matter of multiplication and division such that we get the desired unit. Let's start with our specific heat 0.245 J/(K g) And let's cancel out the g term. Multiplying by our g term will do nicely. So 0.245 J/(K g) * 350 g = 85.75 J/K Now let's cancel out the K term. Another multiplication will do it. 85.75 J/K * 107 K = 9175.25 J Since we only have 3 significant figures in our data, round to 3 significant figures, giving 9180 J.

Answer 2

Specific heat equation is given as

`Q =m* c* \Delta T`

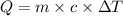
where, Q = energy required

m = mass

c = specific heat

`\Delta T` = change in temperature

Specific heat of silver (
`Ag`) is
`0.245 J/^(o)C g`

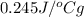
Mass of silver = 350 g

`\Delta T =T_(final)-T_(intital)`

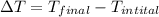
Convert 400 K to degree Celsius = 400-273 = 127 K

Convert 293 K to degree Celsius = 293-273 = 20 K

Thus,
`\Delta T =127 K-20 K`

=
`107^(o)C`

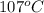
Put the values,

`Q =m* c* \Delta T`

`Q = 350 g* 0.245 J/^(o)C g*(107^(o)C)`

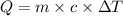
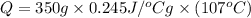
= 9175.25 J

Hence, energy required to raise the temperature is 9175.25 J