Question

Which is the correct simplified form of the expression x^1/2y^-1/3/x^1/4y^1/2

Answer 1

`\bf \left.\qquad \qquad \right.\textit{negative exponents}\\\\ a^{-{ n}} \implies \cfrac{1}{a^( n)} \qquad \qquad \cfrac{1}{a^( n)}\implies a^{-{ n}} \qquad \qquad a^{{{ n}}}\implies \cfrac{1}{a^{-{{ n}}}}\\\\ -------------------------------\\\\`


`\bf \cfrac{x^{(1)/(2)}y^{-(1)/(3)}}{x^{(1)/(4)}y^{(1)/(2)}}\implies \cfrac{x^{(1)/(2)}x^{-(1)/(4)}}{y^{(1)/(2)}y^{(1)/(3)}}\implies \cfrac{x^{(1)/(2)-(1)/(4)}}{y^{(1)/(2)+(1)/(3)}}\implies \cfrac{x^{(2-1)/(4)}}{y^{(3+2)/(6)}}\implies \cfrac{x^{(1)/(4)}}{y^{(5)/(6)}}`

Answer 2

(A)
`\frac{x^{(1)/(4)}}{y^{(5)/(6)}}`

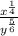
Explanation:

The given expression is:

`\frac{x^{(1)/(2)}y^{(-1)/(3)}}{x^{(1)/(4)}y^{(1)/(2)}}`

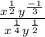
Upon solving the given expression, we get

=
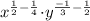`{x^{(1)/(2)-(1)/(4)}{\cdot}}{y^{(-1)/(3)-(1)/(2)}}` (using the property of exponents and powers that if base is same then the powers gets added.)

=
`x^{(1)/(4)}{\cdot}y^{(-5)/(6)}`

=
`\frac{x^{(1)/(4)}}{y^{(5)/(6)}}`

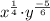
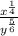
which is the required simplified form of the given equation.

Hence, option A is correct.