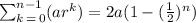The formula for a geometric sequence is:
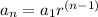
where a_1 is the first term of a sequence and r is the "coomon ratio"
We can see that theach term is half the previous term, thus r = 1/2
We can write:
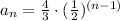
Now, that we know the sequence formula, we can write the sum of the sequence:
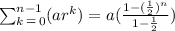
Thus: