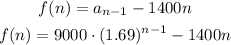Question

Hello! Just want to confirm my answers, the rubric is linked below as well. Thank you!

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Write the given equation for explicit formula

`\begin{gathered} a_n=a_1\cdot r^(n-1) \\ where\text{ }a_1\text{ is the initial count\lparen first term\rparen} \\ r\text{ is the common ratio} \\ n\text{ is the number of years} \end{gathered}`

STEP 2: Write the given details

`\begin{gathered} a_1=9000 \\ r=1+(69)/(100)=1.69\text{ since it is a growth rate} \\ \\ Hence,the\text{ equation is given as:} \\ a_x=9000(1.69)^(x-1) \end{gathered}`

STEP 3: Get the explicit equation for f(n)

n = x

Substitute n for x in the equation in step 2.

Therefore, the explicit equation is given as:

`f(n)=9000\cdot(1.69)^(n-1)`

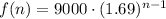
STEP 4: Answer part B

To get how many lionfish in the bay after 6 years

`\begin{gathered} From\text{ equation above,} \\ n=6 \\ f(6)=9000\cdot(1.69)^(6-1) \\ f(6)=9000\cdot1.69^5 \\ f(6)=9000\cdot13.78584918 \\ f(6)=124072.6427 \\ f(6)\approx124073 \end{gathered}`

Hence, there will be approximately 124073 lionfish

STEP 5: Get the recursive formula

1400 lionfish was removed per year, this gives an equation defined below:

Recursive formula is given as

`a_n=r(a_(n-1))`

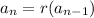
Since we know that the difference each year is 1400, this gives the equation below:

`a_n-1400`

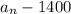
By substitution, the recursive formula will be given by:

Since 1400 is removed each year, we have:

`\begin{gathered} f(n)=a_(n-1)-1400n \\ f(n)=9000\cdot(1.69)^(n-1)-1400n \end{gathered}`