Question

Find the second degree Taylor polynomial for f(x)= sqrt(x^2+8) at the number x=1

Answer 1

`\displaystyle P_2(x) = 3 + (1)/(3)(x - 1) + (4)/(27)(x - 1)^2`

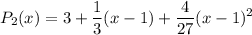
General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Algebra I

Functions

• Function Notation

Calculus

Differentiation

• Derivatives
• Derivative Notation

Derivative Property [Addition/Subtraction]:
`\displaystyle (d)/(dx)[f(x) + g(x)] = (d)/(dx)[f(x)] + (d)/(dx)[g(x)]`

Basic Power Rule:

1. f(x) = cxⁿ
2. f’(x) = c·nxⁿ⁻¹

Derivative Rule [Chain Rule]:
`\displaystyle (d)/(dx)[f(g(x))] =f'(g(x)) \cdot g'(x)`

Taylor Polynomials

• Approximating Transcendental and Elementary Functions
• 
  `\displaystyle P_n(x) = (f(c))/(0!) + (f'(c))/(1!)(x - c) + (f''(c))/(2!)(x - c)^2 + (f'''(c))/(3!)(x - c)^3 + ... + (f^n(c))/(n!)(x - c)^n`

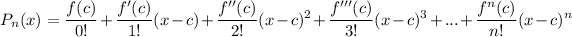
Explanation:

*Note: I will not be showing the work for derivatives as it is relatively straightforward. If you request for me to show that portion, please leave a comment so I can add it. I will also not show work for elementary calculations.

Step 1: Define

Identify

f(x) = √(x² + 8)

Center: x = 1

n = 2

Step 2: Differentiate

1. [Function] 1st Derivative:
   `\displaystyle f'(x) = (x)/(√(x^2 + 8))`
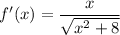
2. [Function] 2nd Derivative:
   `\displaystyle f''(x) = \frac{8}{(x^2 + 8)^\bigg{(3)/(2)}}`

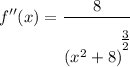
Step 3: Evaluate

1. Substitute in center x [Function]:
   `\displaystyle f(1) = √(1^2 + 8)`
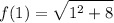
2. Simplify:
   `\displaystyle f(1) = 3`
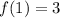
3. Substitute in center x [1st Derivative]:
   `\displaystyle f'(1) = (1)/(√(1^2 + 8))`
4. Simplify:
   `\displaystyle f'(1) = (1)/(3)`
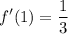
5. Substitute in center x [2nd Derivative]:
   `\displaystyle f''(1) = \frac{8}{(1^2 + 8)^\bigg{(3)/(2)}}`
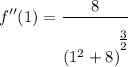
6. Simplify:
   `\displaystyle f''(1) = (8)/(27)`

Step 4: Write Taylor Polynomial

1. Substitute in derivative function values [Taylor Polynomial]:
   `\displaystyle P_2(x) = (3)/(0!) + ((1)/(3))/(1!)(x - c) + ((8)/(27))/(2!)(x - c)^2`
2. Simplify:
   `\displaystyle P_2(x) = 3 + (1)/(3)(x - c) + (4)/(27)(x - c)^2`
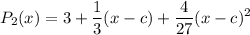
3. Substitute in center c:
   `\displaystyle P_2(x) = 3 + (1)/(3)(x - 1) + (4)/(27)(x - 1)^2`

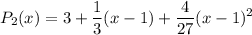
Topic: AP Calculus BC (Calculus I + II)

Unit: Taylor Polynomials and Approximations

Book: College Calculus 10e