Question

Find the slope of a line parallel to the line through the given points. P(-2, 4), Q(6, -2) 4/3 3/4 -3/4

Answer 1

Attached the solution and work.

Answer 2

Option 3 -
`m=-(3)/(4)`

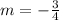
Explanation:

Given : P(-2, 4), Q(6, -2)

To find : The slope of a line parallel to the line through the given points?

Solution :

First we find the slope of the line passes through given points P(-2, 4), Q(6, -2)

The slope formula is

`m=(y_2-y_1)/(x_2-x_1)`

`m=(-2-4)/(6-(-2))`

`m=(-6)/(8)`

`m=-(3)/(4)`

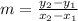
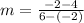
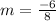
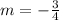
When two lines are parallel then there slope are equal.

Therefore, The slope of a line parallel to the line through the given points is

`m=-(3)/(4)`

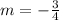
So, Option 3 is correct.