Question

What is the sum of the first eight terms of a geometric series whose first term is 3 and whose common ratio is 1/2?

Answer 1

The answer to this question What is the sum of the first eight terms of a geometric series whose first term is 3 and whose common ratio is 1/2 is (7/65/128)

Answer 2

`(765)/(128)`

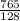
Explanation:

We have been given that 1st term of a geometric series is 3 and common difference is 1/2 . We are asked to find the sum of 1st 8 terms of the given series.

We will use geometric series formula to solve our given problem.

`S_n=(a_1(1-r^n))/(1-r)`

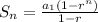
Upon substituting our given values in the above formula we will get,

`S_8=(3(1-((1)/(2))^8))/(1-(1)/(2))`

`S_8=(3(1-(1^8)/(2)^8))/((2-1)/(2))`

`S_8=(3(1-(1)/(256)))/((1)/(2))`

`S_8=(3((256-1)/(256)))/((1)/(2))`

`S_8=(3((255)/(256)))/((1)/(2))`

`S_8=3((255)/(256)*(2)/(1))`

`S_8=3((255)/(128))`

`S_8=(765)/(128)`

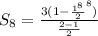
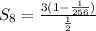
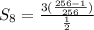
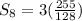
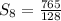
Therefore, the sum of our given series is
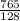`(765)/(128)`.