Question

How do I go about solving sin π/4 + cos π/4

Answer 1

Hello Shakyiagrooms. We know:


`\sin((\pi)/(4)) = (1)/(√(2))`
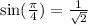
AND

`\cos((\pi)/(4)) = (1)/(√(2))`

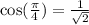
By knowing this, we can set the problem up as the following:

`(1)/(√(2)) + (1)/(√(2))`
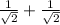
Now we can add them since they have the same denominators:

`(1)/(√(2)) +(1)/(√(2)) = (2)/(√(2))`
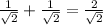
Now we have to rationalize the radical denominator
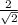
`(2)/(√(2))`. We do this by multiplying
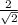
`(2)/(√(2))` by
`(√(2))/(√(2))`


`(2)/(√(2))`

`(2)/(√(2)) * (√(2))/(√(2)) = (2√(2))/(√(4))`

`(2√(2))/(√(4)) = (2√(2))/(2) = √(2)`
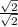
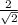
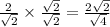
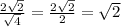
So our answer "Drum Roll Please" =

`\sin((\pi)/(4)) + \cos((\pi)/(4)) = √(2)`